Neural field theories
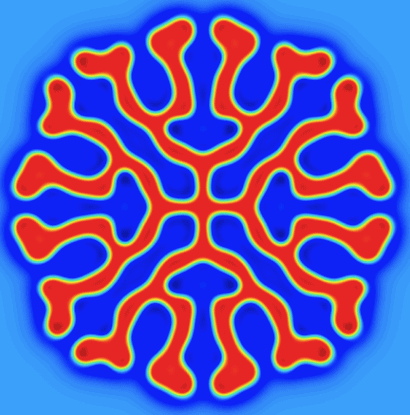Experimental studies have revealed the propagation of travelling bursts of activity in cortical and thalamic brain slices. Experiments in primates have also revealed that prefrontal cortical networks can support spatially localized areas of high activity, thought to be important for the functioning of working memory. Such waves and bumps of firing rate activity are a consequence of non-local synaptic interactions and the intrinsic behavior of local neuronal circuitry. The mathematical description of synaptically coupled neural tissue typically involves the use of integral equations. Apart from a spatial integral mixing the network connectivity function with space-dependent delays, arising from non-instantaneous axonal communication, these integral models can also include a temporal integration over some appropriately identified distributed delay kernel. These distributed delay kernels are biologically motivated and represent the response of biological synapses to spiking inputs. I am interested in using techniques from dynamical systems theory to study waves and patterns in such integral neural field models. This work is being done in collaboration with Gabriel Lord, Markus Owen, Carlo Laing and Daniele Avitabile. Moreover, network studies that include a description of the slow T-type calcium current are known to support non-smooth waves of a type that can lurch through a medium. This work is highly relevant to modelling thalamic tissue and the emergence of EEG sleep rhythms in thalamo-cortical networks.
Scholarpedia entry on Neural Fields.
Relevant publications:
| S Coombes G J Lord and M R Owen 2003 Waves and bumps in neuronal networks with axo-dendritic synaptic interactions, Physica D, Vol 178, 219-241 |
| S Coombes and M R Owen 2004 Evans functions for integral neural field equations with Heaviside firing rate function, SIAM Journal on Applied Dynamical Systems, Vol 34, 574-600 |
| S Coombes 2005 Waves and bumps in neural field theories, Biological Cybernetics, Vol 93, 91-108 |
| S Coombes and M R Owen 2005 Bumps, breathers, and waves in a neural network with spike frequency adaptation, Physical Review Letters, 94, 148102. |
| S Coombes and M R Owen 2007 Exotic dynamics in a firing rate model of neural tissue with threshold accommodation, AMS Contemporary Mathematics, Vol 440 "Fluids and Waves: Recent Trends in Applied Analysis", (Ed. Fernanda Botelho, Thomas Hagen, and James Jamison), 123-144 |
| N A Venkov, S Coombes and P C Matthews 2007 Dynamic instabilities in scalar neural field equations with space-dependent delays, Physica D, Vol 232, 1-15 |
| M R Owen, C R Laing and S Coombes 2007 Bumps and rings in a two-dimensional neural field: splitting and rotational instabilities, New Journal of Physics, Vol 9, 378 |
| S Coombes, N A Venkov, L Shiau, I Bojak, D T J Liley and C R Laing 2007 Modeling electrocortical activity through improved local approximations of integral neural field equations, Physical Review E, Vol 76, 051901-8. |
| S Coombes and H Schmidt 2010 Neural fields with sigmoidal firing rates: approximate solutions, Discrete and Continuous Dynamical Systems Series A, Vol 28, 1369 - 1379 |
| S Coombes 2010 Large-scale neural dynamics: Simple and complex, NeuroImage, Vol 52, 731–739 |
| S Coombes and C R Laing 2011 Pulsating fronts in periodically modulated neural field models, Physical Review E, Vol 83, 011912 |
| S Coombes, H Schmidt and I Bojak 2012 Interface dynamics in planar neural field models, Journal of Mathematical Neuroscience, 2:9. |
| P C Bressloff and S Coombes 2013 Neural `bubble' dynamics revisited, Cognitive Computation, Vol 5(3), 281-294 |
| H G E Meijer and S Coombes 2014 Travelling waves in a neural field model with refractoriness, Journal of Mathematical Biology, Vol 68(5), 1249-1268 |
| S Coombes, H Schmidt and D Avitabile 2014 Spots: Breathing, drifting and scattering in a neural field model, Neural Fields, Ed. S Coombes, P beim Graben, R Potthast and J J Wright, Springer Verlag |
| S Coombes, P beim Graben and R Potthast 2014 Tutorial on Neural Field Theory, Neural Fields, Ed. S Coombes, P beim Graben, R Potthast and J J Wright, Springer Verlag |
| H G E Meijer and S Coombes 2014 Travelling waves in models of neural tissue: from localised structures to periodic waves, EPJ Nonlinear Biomedical Physics, Vol 2:3 |
| R Thul, S Coombes and C R Laing 2016 Neural field models with threshold noise, Journal of Mathematical Neuroscience, 6:3 |
| S Visser, R Nicks, O Faugeras and S Coombes, 2017 Standing and travelling waves in a spherical brain model: the Nunez model revisited, Physica D, Vol 349, 27–45 |